Skip over navigation
Key question
We know the small circle rolls around inside the big one. What can we say about arc lengths?
Possible support
Learners might draw their own interactive diagrams using Geogebra.
Ask about how far a bicycle goes forward when the wheels rotate through exactly one revolution.
What about a revolution by a given angle?
What if the road was curved?
Possible extension
See the problem Illusion.
Just Rolling Round
Age 14 to 16
Challenge Level 
 |
Why do this problem?
In this
problem , the interactivity enables learners to experiment and
make their own conjectures about the locus. The proof requires only
simple geometrical reasoning involving circle theorems and arc
lengths.
Possible approach
Learners might first experiment with the interactivity and
make a conjecture about the locus, then try to prove their
conjecture.
|
Key question
We know the small circle rolls around inside the big one. What can we say about arc lengths?
Possible support
Learners might draw their own interactive diagrams using Geogebra.
Ask about how far a bicycle goes forward when the wheels rotate through exactly one revolution.
What about a revolution by a given angle?
What if the road was curved?
Possible extension
See the problem Illusion.
You may also like
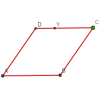
Roaming Rhombus
We have four rods of equal lengths hinged at their endpoints to form a rhombus ABCD. Keeping AB fixed we allow CD to take all possible positions in the plane. What is the locus (or path) of the point D?
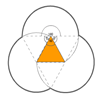
Triangles and Petals
An equilateral triangle rotates around regular polygons and produces an outline like a flower. What are the perimeters of the different flowers?
