Skip over navigation
Jack and Ashley from Sir Harry Smith Community College sent us the following thoughts:
(1+2+3+...+n)^2 is equal to 1^3+2^3+3^3+...+n^3, which can also be written \left(\sum_{r=1}^n r \right)^2 = \sum_{r=1}^n r^3
We tested this formula with two examples:
1^3+2^3+...+6^3=441, and 1+2+...+6=21, 21^2=441.
1^3+2^3+...+10^3=3025, and 1+2+...+10=55, 55^2=3025.
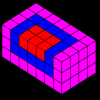
An anonymous solver from Mearns Castle School explained very clearly how the diagram shows the two parts of the formula:
The area of the square can be looked at in different ways.
The columns are 1 then 2 then 3 then 4 units long. It is a square, so the area is the length squared which is (1+2+3+4+5+6)^2.

Now look at each of the 6 backward L shapes.
We have the indicated square on the diagonal (for example, on the blue L shape 5^2), then we have other congruent squares (shaded in the same colour).
Note that in even levels, there are two half-squares which make up one full square. So the area of the blue shaded level is: 5^2+4\times5^2 = (5^2)(1+4) =(5^2)(5) =5^3
As this is true for the first, second, third... n^{th} level of the area of the n^{th} level is n^3.
This means if there are n levels, the length of one side of the square is 1+2+3...+(n-1)+n and thus the area equals (1+2+3...+(n-1)+n)^2.
But considering the area in terms of each level gives (1^3)+(2^3)+(3^3)...+(n-1)^3+(n^3).
So it can be said (1^3)+(2^3)+(3^3)...+(n-1)^3+(n^3) = (1+2+3...+(n-1)+n)^2.
John from Takapuna Grammar School used induction to prove the formula:
Using induction we should first prove that 1^3 is equal to 1^2, which is obvious, as they're both equal to 1.
Now to prove that any added number (n+1) would keep the equation satisfied:
The formula for any triangular number (1 + 2 + 3...+ n) is \frac{n(n+1)}{2}.
Now we observe the way in which new tiles are put on. There are (n+1) squares to be added, which contain (n+1)^2 units each, making (n+1)^3.
One of these squares is placed diagonally to the original square and the other n squares are placed along the sides.
Since there are two sides to place against, this means that there are \frac{n}{2} squares per side, and because each square is (n+1) units long that means there are \frac{n(n+1)}{2} units covered on each side.
There are \frac{n(n+1)}{2} units on each side of the original square so that means all of the units on each side are covered, leaving the last (n+1)^2 square (the one that is diagonal to the original square) to make a new square itself.
Finally, we know that each side of this new square is the (n+1)^{th} triangular number, because the previous square's length was the n^{th} triangular number, and this length has increased by (n+1).
Therefore the equation (1^3 + 2^3...n^3)=(1 + 2... + n)^2 holds for all positive whole numbers, because it is true for n=1 and if it's true for any number n then it's true for n + 1.

Or search by topic
Number and algebra
Geometry and measure
Probability and statistics
Working mathematically
Advanced mathematics
For younger learners
Picture Story
Age 14 to 16
Challenge Level 





- Problem
- Getting Started
- Student Solutions
- Teachers' Resources
Jack and Ashley from Sir Harry Smith Community College sent us the following thoughts:
(1+2+3+...+n)^2 is equal to 1^3+2^3+3^3+...+n^3, which can also be written \left(\sum_{r=1}^n r \right)^2 = \sum_{r=1}^n r^3
We tested this formula with two examples:
1^3+2^3+...+6^3=441, and 1+2+...+6=21, 21^2=441.
1^3+2^3+...+10^3=3025, and 1+2+...+10=55, 55^2=3025.
An anonymous solver from Mearns Castle School explained very clearly how the diagram shows the two parts of the formula:
The area of the square can be looked at in different ways.
The columns are 1 then 2 then 3 then 4 units long. It is a square, so the area is the length squared which is (1+2+3+4+5+6)^2.

Now look at each of the 6 backward L shapes.
We have the indicated square on the diagonal (for example, on the blue L shape 5^2), then we have other congruent squares (shaded in the same colour).
Note that in even levels, there are two half-squares which make up one full square. So the area of the blue shaded level is: 5^2+4\times5^2 = (5^2)(1+4) =(5^2)(5) =5^3
As this is true for the first, second, third... n^{th} level of the area of the n^{th} level is n^3.
This means if there are n levels, the length of one side of the square is 1+2+3...+(n-1)+n and thus the area equals (1+2+3...+(n-1)+n)^2.
But considering the area in terms of each level gives (1^3)+(2^3)+(3^3)...+(n-1)^3+(n^3).
So it can be said (1^3)+(2^3)+(3^3)...+(n-1)^3+(n^3) = (1+2+3...+(n-1)+n)^2.
John from Takapuna Grammar School used induction to prove the formula:
Using induction we should first prove that 1^3 is equal to 1^2, which is obvious, as they're both equal to 1.
Now to prove that any added number (n+1) would keep the equation satisfied:
The formula for any triangular number (1 + 2 + 3...+ n) is \frac{n(n+1)}{2}.
Now we observe the way in which new tiles are put on. There are (n+1) squares to be added, which contain (n+1)^2 units each, making (n+1)^3.
One of these squares is placed diagonally to the original square and the other n squares are placed along the sides.
Since there are two sides to place against, this means that there are \frac{n}{2} squares per side, and because each square is (n+1) units long that means there are \frac{n(n+1)}{2} units covered on each side.
There are \frac{n(n+1)}{2} units on each side of the original square so that means all of the units on each side are covered, leaving the last (n+1)^2 square (the one that is diagonal to the original square) to make a new square itself.
Finally, we know that each side of this new square is the (n+1)^{th} triangular number, because the previous square's length was the n^{th} triangular number, and this length has increased by (n+1).
Therefore the equation (1^3 + 2^3...n^3)=(1 + 2... + n)^2 holds for all positive whole numbers, because it is true for n=1 and if it's true for any number n then it's true for n + 1.
You may also like
Sums of Powers - A Festive Story
A story for students about adding powers of integers - with a festive twist.
Summing Squares
Discover a way to sum square numbers by building cuboids from small cubes. Can you picture how the sequence will grow?

