Skip over navigation
For many applications mathematicians are not concerned about the exact numerical values of functions but, rather, their behaviour for large values of the variable. Why? One important reason is in the generation of algorithms for implementation on computers: it is often possible to relate the time that the algorithm will take to complete in terms of a simple mathematical function of the number of variables going into the algorithm. Algorithms which take too long to complete will be practically useless, although they may be theoretically beautiful.
An example is the following test, discovered in the 18th century by John Wilson, to see if a number is prime or not:
p is a prime number if and only if (p-2)! = 1mod p
Try using this and you will discover that it is highly inefficient as a way of actually testing for primality, although it is a very beautiful result mathematically.
How Does Your Function Grow?
Age 16 to 18
Challenge Level 
For many applications mathematicians are not concerned about the exact numerical values of functions but, rather, their behaviour for large values of the variable. Why? One important reason is in the generation of algorithms for implementation on computers: it is often possible to relate the time that the algorithm will take to complete in terms of a simple mathematical function of the number of variables going into the algorithm. Algorithms which take too long to complete will be practically useless, although they may be theoretically beautiful.
An example is the following test, discovered in the 18th century by John Wilson, to see if a number is prime or not:
p is a prime number if and only if (p-2)! = 1mod p
Try using this and you will discover that it is highly inefficient as a way of actually testing for primality, although it is a very beautiful result mathematically.
You may also like
Climbing Powers
$2\wedge 3\wedge 4$ could be $(2^3)^4$ or $2^{(3^4)}$. Does it make any difference? For both definitions, which is bigger: $r\wedge r\wedge r\wedge r\dots$ where the powers of $r$ go on for ever, or $(r^r)^r$, where $r$ is $\sqrt{2}$?
Little and Large
A point moves around inside a rectangle. What are the least and the greatest values of the sum of the squares of the distances from the vertices?
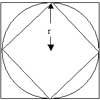
Approximating Pi
By inscribing a circle in a square and then a square in a circle find an approximation to pi. By using a hexagon, can you improve on the approximation?