Power Countdown

Power Countdown printable sheet

In the game of Power Countdown, you use a set of numbers to make a target number, but unlike the usual Countdown game where you can use $+, -, \times$ or $\div$, the only operations you can use are raising a number to a power, taking the reciprocal of a number, or finding the product of two numbers.

Each number can only be used once. You don't have to use all the numbers. There is often more than one way of making a particular target, so see how many different ways you can find.

Watch the video to see some examples.

Can you find any other ways of making $8$?
Are there any ways which use all the numbers?

Here is another selection.


How many ways are there to make the target number of $125$?


Below is a selection of numbers and five targets.



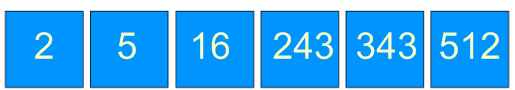
How many different ways can you find to make each target?

Are there any targets you can't make? How close can you get?