Skip over navigation
Weird Universes
Age 16 to 18
Challenge Level 
The first diagram represents a torus. Shoot along any direction.
In the second one, shoot diagonally right and up.
The third diagram is like a pyramid teabag! Shoot diagonally up and left.
In the fourth diagram, shoot straight to the right.
The first and third diagrams can be created out of a sheet of paper.
You may also like
Euler's Formula
Some simple ideas about graph theory with a discussion of a proof of Euler's formula relating the numbers of vertces, edges and faces of a graph.
Air Nets
Can you visualise whether these nets fold up into 3D shapes? Watch the videos each time to see if you were correct.
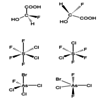
Stereoisomers
Put your visualisation skills to the test by seeing which of these molecules can be rotated onto each other.