Skip over navigation
J Darcy from Pulner Junior School and Otto completed the first Factor Track in the following 9 moves:
Divide 60 by 2 and move on 2 spaces, then divide 14 by 7 and move on 7 spaces. 7 is a factor of 28 so move on 7 spaces. 18 divides into 3 so move on 3 spaces. 14 divides into 2 so move on 2 spaces. 24 divides into 3 so move to 36. 36 divides into 3 to move to 32. 32 divides by 4 so move to 14. 14 divides by 7 to reach the end!
Lots of you went straight to the more challenging one and managed it in 10 moves including students from Wilson's School, St Helen's C of E Primary School, Baston C of E Primary School, MacDiarmid Primary School and Highfield School.
Ultra Violet Class from Unicorn explain their solution here:
Issy, Laura and Anna worked as a team to solve this problem. They found that doing the training track gave them a very good understanding of the problem. On the more complicated track they found the shortest route involved 10 steps. They used a counter and recorded each step by writing the sequence of numbers linked by arrows with the number of moves written above:
60 (3 moves) 48 (6 moves) 28 (7 moves) 51 (3 moves) 96 (4 moves) 81 (3 moves) 54 (2 moves) 87 (3 moves) 72 (4 moves) 49 (7 moves) END
They noted that the only point they could not go from corner to corner was on the first row where they had to use 2 moves between the numbers but there were lots of options:
60 to 14 to 28
60 to 48 to 28
60 to 25 to 28
60 to 45 to 28
They found using divisibility rules to check for factors of the larger numbers a very useful approach. The most helpful one being the divisibility rule for the number 3.
Alex, Jack and Jamie also came up with the shortest route of 10 steps. Peter, Ben and Jack all tried going the longer route and found they could do it in 18, 17 and 16 steps. The shortest route of 16 steps was achieved by moving from corner to corner in all but two cases. This was the first row as described above and the very last column where they had to go 12 to 49 to END.
Mr Bouchard's Class from Richmon Elementary School, USA, sent us a picture of their solution:
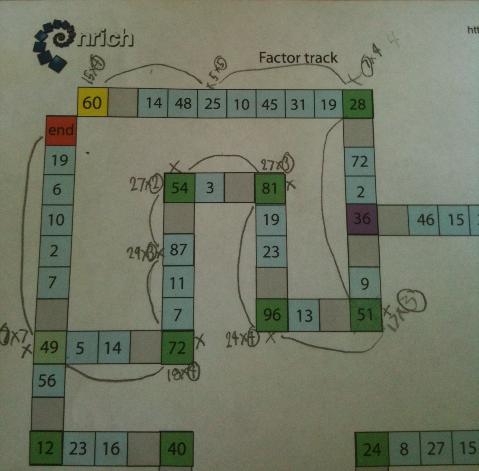
And Krystov's explanation can be found here.
Students from Greenacre School for Girls decided to make their own Factor Tracks. See if you can complete them.
1 by Erica and Helena
2 by Grace and Katie
3 by Rebecca and Elizabeth
4 by Rosie
5 by Sophie, Esme and Josie
6 by Zoe and Rachel
Factor Track
Age 7 to 11
Challenge Level 
J Darcy from Pulner Junior School and Otto completed the first Factor Track in the following 9 moves:
Divide 60 by 2 and move on 2 spaces, then divide 14 by 7 and move on 7 spaces. 7 is a factor of 28 so move on 7 spaces. 18 divides into 3 so move on 3 spaces. 14 divides into 2 so move on 2 spaces. 24 divides into 3 so move to 36. 36 divides into 3 to move to 32. 32 divides by 4 so move to 14. 14 divides by 7 to reach the end!
Lots of you went straight to the more challenging one and managed it in 10 moves including students from Wilson's School, St Helen's C of E Primary School, Baston C of E Primary School, MacDiarmid Primary School and Highfield School.
Ultra Violet Class from Unicorn explain their solution here:
Issy, Laura and Anna worked as a team to solve this problem. They found that doing the training track gave them a very good understanding of the problem. On the more complicated track they found the shortest route involved 10 steps. They used a counter and recorded each step by writing the sequence of numbers linked by arrows with the number of moves written above:
60 (3 moves) 48 (6 moves) 28 (7 moves) 51 (3 moves) 96 (4 moves) 81 (3 moves) 54 (2 moves) 87 (3 moves) 72 (4 moves) 49 (7 moves) END
They noted that the only point they could not go from corner to corner was on the first row where they had to use 2 moves between the numbers but there were lots of options:
60 to 14 to 28
60 to 48 to 28
60 to 25 to 28
60 to 45 to 28
They found using divisibility rules to check for factors of the larger numbers a very useful approach. The most helpful one being the divisibility rule for the number 3.
Alex, Jack and Jamie also came up with the shortest route of 10 steps. Peter, Ben and Jack all tried going the longer route and found they could do it in 18, 17 and 16 steps. The shortest route of 16 steps was achieved by moving from corner to corner in all but two cases. This was the first row as described above and the very last column where they had to go 12 to 49 to END.
Mr Bouchard's Class from Richmon Elementary School, USA, sent us a picture of their solution:
And Krystov's explanation can be found here.
Students from Greenacre School for Girls decided to make their own Factor Tracks. See if you can complete them.
1 by Erica and Helena
2 by Grace and Katie
3 by Rebecca and Elizabeth
4 by Rosie
5 by Sophie, Esme and Josie
6 by Zoe and Rachel
You may also like
Area and Perimeter
What can you say about these shapes? This problem challenges you to create shapes with different areas and perimeters.