Pattern Sniffing

What do young children understand as pattern?  As Merilyn Buchanan points out in her article Pattern Power, when asked "What is pattern?", young children often give examples of visual patterns on wallpaper, wrapping paper, clothes etc.  The key element tends to be the idea of a repeat.  In analysing whether there is a pattern or not, learners look for similarities and differences, and this is true when considering number pattern too. 

What do we mean by pattern in number?
In order for children to identify number patterns, they need to have lots of experience of playing with numbers.  One important element of this number play is having opportunities to represent numbers in different ways.  This could be as dots as on dice or dominoes, dots on a grid or using other practical equipment such as Cuisenaire rods.  For example, we can grasp why 25 is a 'square' number more easily if we create representations of 5 and 25: 

 

 

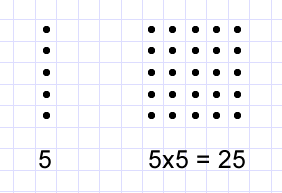
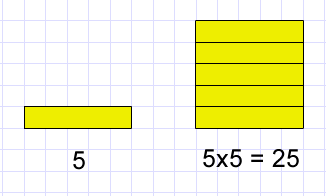
These different representations help to build children's mental images of number and to reveal the underlying structure of our number system. Learners with a secure understanding of, for example, the 'twoness' of two, will find it easier to distinguish what is the same and what is different about a selection of numbers.

Having had this hands-on experience of number play, we might expect young children to begin to recognise number sequences, to know what becomes before and to be able to continue a sequence.  At a more sophisticated level, children can then go on to find the general term.  When considering number pattern, we might immediately think of sequences of evens, odds, square numbers, triangular numbers etc. but of course exploring patterns in times tables is invaluable.

Why is being able to identify number patterns useful?
As intimated above, having experience of patterns within our number system helps to embed a deeper understanding of that number system.  This experience not only comes from playing with numbers, as already mentioned, but also from tackling problems.  At NRICH, one of our aims is for children to think like mathematicians so that they approach a new problem with confidence.  Cuoco, Goldenberg and Mark (1996) suggest eight habits of mind characteristic of mathematicians, so learners should be:

Relevant to this article is 'pattern sniffers'.  You may well have experienced yourself the delight that comes with finding a pattern as you work on a problem.  We can try to foster this sense of wonder in our learners, but why is being a 'pattern sniffer' so important?  Identifying a pattern often means we save time and that can be satisfying.  However, there is a danger that pattern spotting in itself becomes the focus of activity.  Once a pattern has been identified, the next question should be 'why does this pattern occur?'.  By trying to answer 'why?', learners will gain greater insight into mathematical structures and therefore further their conceptual understanding.

How can NRICH help?
In this Number Patterns feature we have chosen a range of activities which offer children the chance to create, look for and explain patterns. 

Domino Patterns (Stage 1) is an open task, encouraging children to explore the structure of a set of dominoes in their own way and to think about ways of constructing different patterns.  Domino Sets (Stage 2) extends the idea of pattern in the same domino context.  This time, children are challenged to find a way of working out whether a set of dominoes is complete or not.

In Ring a Ring of Numbers (Stage 1), learners find lots of examples and then are asked what they have in common.  By probing into the reasons for their observations, children will gain a greater understanding of the properties of odd and even numbers.  In the same way, in Consecutive Numbers (Stage 2), pupils look for similarities and differences between different examples they create.  Again, by trying to explain why the patterns occur, learners will deepen their understanding of the number system.

Lots of Lollies (Stage 1) and Times Tables Shifts (Stage 2) both give children the opportunity to explore patterns in the times tables.  Each of these activities not only helps children to become more familiar with their tables, but also gets to the heart of what times tables really are.

What next?
For further activities, see our related content:  More Number Patterns Lower Primary and More Number Patterns Upper Primary. The secondary feature Patterns in Number Sequences extends these ideas.


References
Cuoco A., Goldenberg E. P. and Mark J. (1996) 'Habits of Mind: An Organizing Principle for Mathematics Curricula'. Journal of Mathematical Behavior 15 375-402 Cuoco_etal-1996.pdf


Here is a PDF version of this article.