Skip over navigation
Consider powers of 1 + x.
If you can't get started investigate the formula for n = 2, 3,4 ...

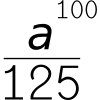
Or search by topic
Number and algebra
Geometry and measure
Probability and statistics
Working mathematically
Advanced mathematics
For younger learners
Binomial
Age 16 to 18
Challenge Level 





- Problem
- Getting Started
- Student Solutions
- Teachers' Resources
Consider powers of 1 + x.
If you can't get started investigate the formula for n = 2, 3,4 ...
You may also like
Telescoping Series
Find S_r = 1^r + 2^r + 3^r + ... + n^r where r is any fixed positive integer in terms of S_1, S_2, ... S_{r-1}.
Remainder Hunt
What are the possible remainders when the 100-th power of an integer is divided by 125?

